Comments:  Thermodynamics is not all about Carnot cycles, gases, and so forth. LD4 gives an interesting application in an unfamiliar setting. Recall that once you have S(E), a temperature T can be defined. Bekenstein actually demonstrated that the area A of the event horizon of a black hole acts like an entropy, always increasing through interactions. Hawking found the correct proportionality between S and A and then demonstrated that a hot black hole must radiate, a result that would not be surprising for a normal hot body, but one contrary to the usual lore that nothing can escape from the black hole. How long would a black hole of your mass last?
		A complete quantum mechanical derivation of S for black holes was been obtained only recently using string theory [A. Strominger and C. Vafa, Phys. Lett. B 379, 99 (1996)]. Strominger showed in addition that information is not lost at the quantum level when something falls into a black hole and its energy reappears as thermal radiation. The information is preserved near the event horizon [A. Strominger, Phys. Rev. Lett. 77, 3498 (1996)].

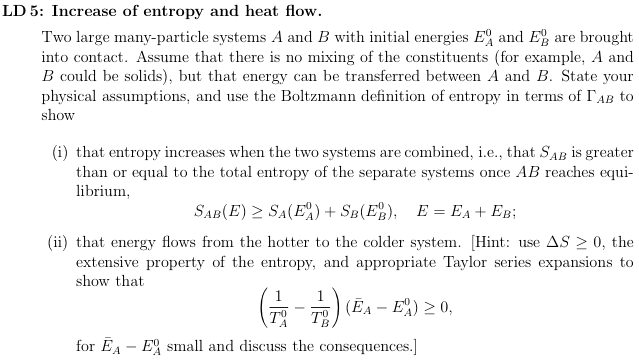
Comments: The results of this problem demonstrate that Boltzmann's entropy has the expected properties of the thermodynamic entropy defined by Clausius. The results can be extended to show that systems in equilibrium have equal chemical potentials, pressures, etc.

Comments: The physical picture here is simple if possible multiparticle overlaps or collisions are neglected. The number of three-particle overlaps at a gas density n = N/V is proportional to n3/3! for N >> 1, so should be negligible relative to the two-particle correction you are asked to calculate provided n << 1 (low density). The only sublety in the calculation is the need to factor out so that you can write the result of the (approximate) volume integration as a ratio of generalized factorials (gamma functions). Your result in this calculation is the hard-sphere version of the Saha-Bose equation of state. When corrected for the effects of longer range interactions, it is significantly better than the van der Waals equation of state with the same correction for gases near the critical point.

Comments: This problem involves the definition of a distribution function in one variable, here the energy E, by "integrating out" all the irrelevant (unobserved) variables. We will encounter other such distributions, e.g., the two-particle Boltzmann momentum distribution, and the two-particle cluster function, in later problems. The calculations are rather simple if you observe that H is just the square p2/2m of a 3N vector in the 3N-dimensional momentum space, use spherical coordinates, integrate over the angles, and then change to the energy as the variable of integration.

FURTHER OPTIONS: