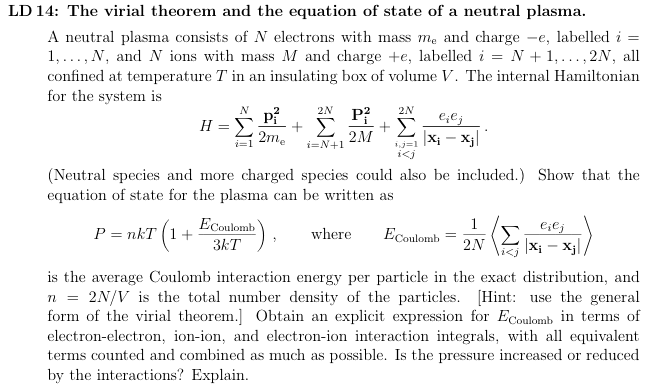
Comment: The virial theorem gives the exact equation of state. You should be able to formulate the result explicitly so that the cancellation between the positive and negative contributions to the Coulomb energy is evident. The result can be expressed in terms of the radial distribution functions for the electrons and ions, that is, the numbers n(r) of like and unlike charges per unit volume a distance r from the charge under consideration. It is clear that these should be equal at sufficiently large distances because the plasma is neutral and distributed uniformly in space. However, explicit calculation of the distribution functions and the Coulomb energy will require the calculation of correlations between particles, for example, in the Debye-Huckel theory we will consider later.

Comment: Part (a) of the problem illustrates the formulation and use of generalized grand distribution functions. Parts (b) and (c) deal with the famous problem of critical opalescence first solved quantitatively by Einstein in 1910. See A. Pais, Subtle is the Lord, a scientific biography of Einstein, p. 100. The solution to the problem corresponds to a mean field treatment of the fluctuations in the liquid-gas phase transition near the critical point, and a calculation gives the mean-field value of a critical exponent, in this case the power with which the compressibility diverges for V --> Vc. We will return to the subject of critical exponents toward the end of the course.

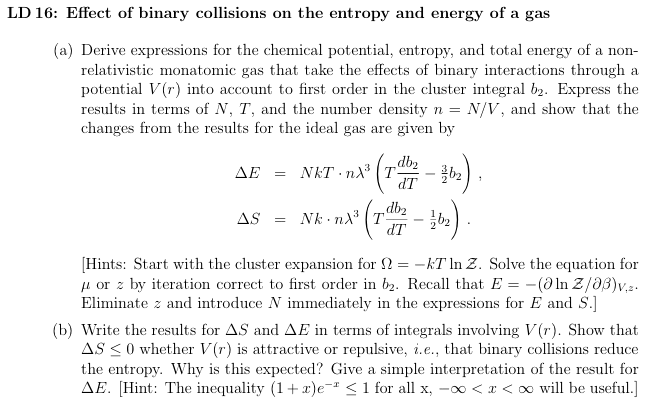
		Comment: This problem extends the calculations with the cluster expansion done in class. You will need to solve for the fugacity z and other quantities as power series in the density n. Iteration will be useful in getting the series. Note that everything should be expanded to a consistent order.
		The result for the energy shift in part (b) has a very simple interpretation in terms of the average potential energy in a two-particle canonical distribution, a generalization of the two-particle Maxwell-Boltzmann distribution used in LD 10 to include the interactions between pairs of particles. Convince yourself of this. The result should also reproduce the perturbation expansion in V/kT for this quantity small.

Comment: This is a typical example of the use of the virial expansion and information about potential shapes in quantum mechanics to get useful information about interatomic interactions. It is oversimplified in the case of helium - quantum effects are significant - but gives fairly accurate information on the location of the potential minimum and depth. It should be clear from your results that you could not get this information by such standard methods as scattering or the measurement of electromagnetic transitions in diatomic bound states.

FURTHER OPTIONS: